Recently, Jiang Xue, a young associate researcher of the Intelligent Medical Ultrasound Innovation Team of Fudan University, collaborated with Dr. Guancong Ma (corresponding author) of Hong Kong Baptist University, Dr. Kun Ding (corresponding author) of Imperial College London, and Professor CT Chan of Hong Kong University of Science and Technology. Important progress has been made in the study of the topological properties of singular points in the system. On November 27, the relevant research results were published in "Science" (https://science.sciencemag.org/content/370/6520/1077). This work was funded by the National Natural Science Foundation of China and the Youth Support Program of the China Association for Science and Technology..
In non-Hermitian systems, branch singularities called Exceptional Points may appear, with non-zero topological charges. The exploration of non-Hermitian physics and the symmetry of odd and even time provides scientists with new ideas for discovering a large number of peculiar physical effects. In this kind of dissipative system, the balance between system gain and loss leads to the appearance of abnormal points or "optimum points" in the parameter space. These abnormal points exist in the equipment parameter space or the material characteristic parameter space. The ability to adjust gain and loss on the characteristics of the series system can cause abnormal arcs.
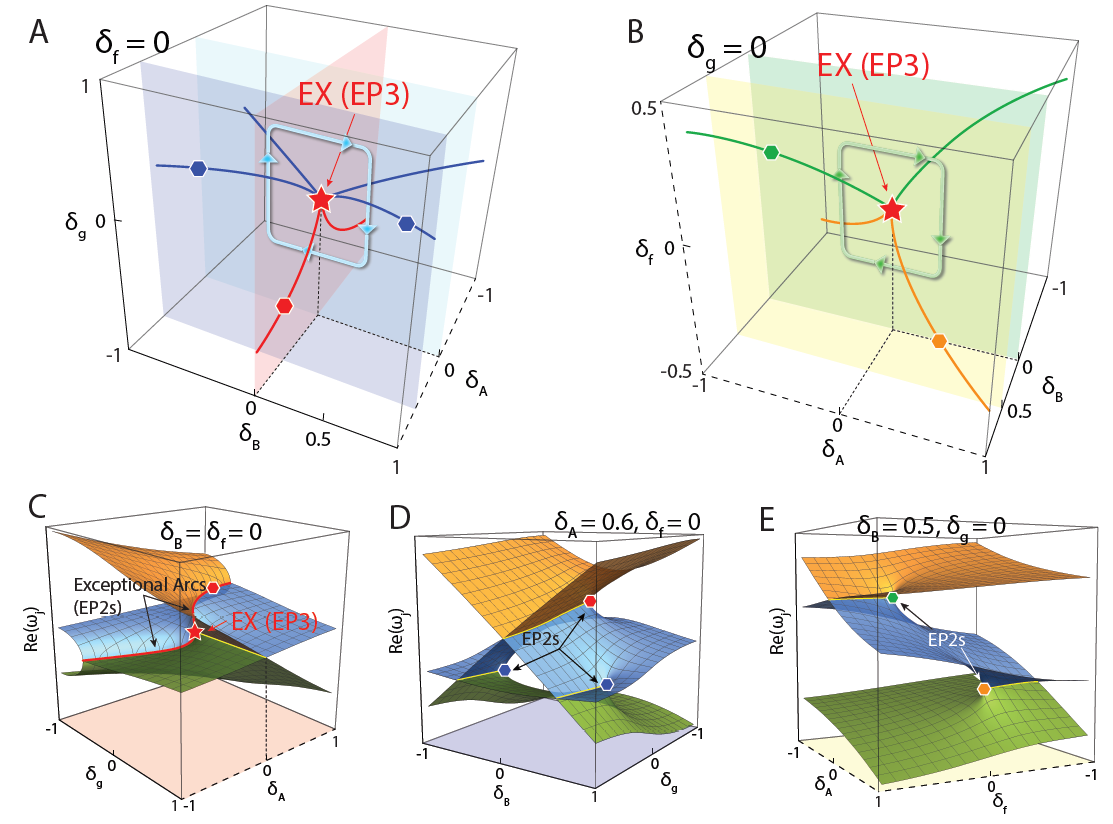
In view of this, researchers such as Ma Guancong, Ding Kun, Jiang Xue and others have designed a brand-new system based on the adjustment of multiple parameters and proved the "Exceptional Nexus" through theoretical and acoustic experiments. It is not only a high-order anomaly. Point, but also the tip singularity of multiple "abnormal arcs". Since the parameter space is divided by "abnormal arcs", the anomalous connections have mixed topology invariance, which consists of different winding numbers related to the Berry phase accumulated on the cyclic paths on different complex planes, and the fractional volume is observed Winding number. By measuring the critical behavior of the wave function, the invariance of mixed topology can be characterized experimentally.
This discovery plays an important role in promoting the basic understanding of non-Hermitian systems and their topologies, and provides a universal way to precisely adjust material parameters and the optimal performance of equipment.
Weiyuan Tang, Xue Jiang, et. al., Exceptional nexus with a hybrid topological invariant, Science, 370, 6250, 2020.